
최단경로 문제
주어진 그래프에서 주어진 두 정점을 연결하는 가장 짧은 경로의 길이를 찾는 문제
- 가중치가 있는 그래프에서의 최단 경로를 찾는 알고리즘을 다룬다.
- 최단 경로를 구성하는 정점들의 목록을 구해주는 것이 아니기 때문에 실제 경로를 계산하기 위해서는 별도의 정보를 저장해야 한다.
- 다양한 그래프의 종류와 특성에 따라 최적화된 많은 최단 경로 알고리즘이 존재한다
음수 간선의 중요성
- 음수 간선을 지나가면 전체 경로의 길이가 짧아진다.
- 음수 사이클이 있을 경우 경로의 길이는 음의 무한대로 발산 할 수 있다.
- 그래서 최단 경로를 찾을 수 없으며 음수 사이클이 존재한다는 것만 확인할 수 있다.
단일 시작점과 모든 쌍의 알고리즘
- 단일 시작점 알고리즘 : 너비 우선 탐색과 비슷하게, 하나의 시작점에서 다른 모든 정점까지 가는 최단 거리 계산
- 모든 쌍 알고리즘 : 모든 정점의 쌍에 대한 최단 거리를 계산
다익스트라의 최단 경로 알고리즘
- 단일 시작점 최단 경로 알고리즘
- 시작 정점 s에서 부터 다른 정점까지의 최단 거리를 계산
우선순위 큐를 사용하는 너비 우선 탐색
- 우선순위 큐에 정점의 번호와 함께 지금까지 찾아낸 해당 정점 까지의 최단 거리를 쌍으로 넣는다.
- 우선순위 큐는 정점까지의 최단 거리를 기준으로 정점을 배열해서 아직 방문하지 않은 정점 중 시작점으로부터의 거리가 가장 가까운 점을 찾는 과정을 간단하게 해준다.
- 각 정점까지의 최단 경로가 갱신될 수 있다.
이 경우 원래 있던걸 그대로 두고 새로운 걸 추가한 뒤 나중에 원래있던게 꺼내지면 무시한다
import java.util.ArrayList;
import java.util.Collections;
import java.util.List;
import java.util.PriorityQueue;
public class Dijkstra {
public void computePath(Vertex sourceVertex) {
sourceVertex.setMinDistance(0);
PriorityQueue<Vertex> priorityQueue = new PriorityQueue<>();
priorityQueue.add(sourceVertex);
while (!priorityQueue.isEmpty()) {
Vertex vertex = priorityQueue.poll();
for (Edge edge : vertex.getEdges()) {
Vertex v = edge.getTargetVertex();
// Vertex u = edge.getStartVertex();
double weight = edge.getWeight();
double minDistance = vertex.getMinDistance() + weight;
if (minDistance < v.getMinDistance()) {
priorityQueue.remove(vertex);
v.setPreviosVertex(vertex);
v.setMinDistance(minDistance);
priorityQueue.add(v);
}
}
}
}
public List<Vertex> getShortestPathTo(Vertex targetVerte) {
List<Vertex> path = new ArrayList<>();
for (Vertex vertex = targetVerte; vertex != null; vertex = vertex.getPreviosVertex()) {
path.add(vertex);
}
Collections.reverse(path);
return path;
}
}다익스트라 알고리즘 동작 과정

다익스트라 알고리즘 시간 복잡도
- 정점마다 인접한 간선을 모두 검사하는 작업 : O(|E|)
- 우선순위 큐에 원소를 넣고 삭제하는 작업 : O(|E| lg |E|)
- O(|E| lg |V|)
벨만-포드의 최단 경로 알고리즘
- 다익스트라 알고리즘은 음수 간선이 있을 경우 정당성이 보장되지 않는다.
- 단일 시작점 최단 경로 알고리즘
- 음수 간선이 있는 그래프에 대해서도 최단 경로를 찾을 수 있다.
- 그래프에 음수 사이클이 있어서 최단 거리가 제대로 정의되지 않을 경우도 알려준다.
- 시작점에서 각 정점까지 가는 최단 거리의 상한을 적당히 예측한 뒤 예측 값과 실제 최단 거리 사이의 오차를 반복적으로 줄여나가는 방식으로 동작한다.
- 수행 과정에서 각 정점까지의 최단 거리의 상한을 담는 배열 upper[]를 유지한다.
벨만-포드의 동작과정
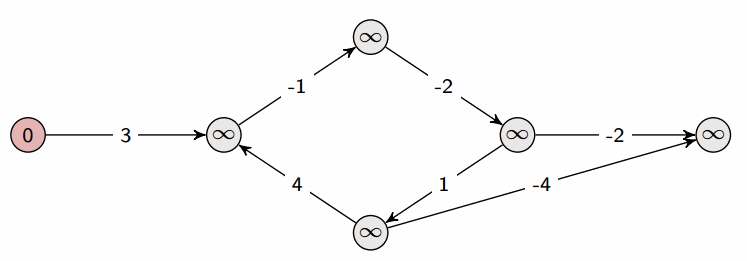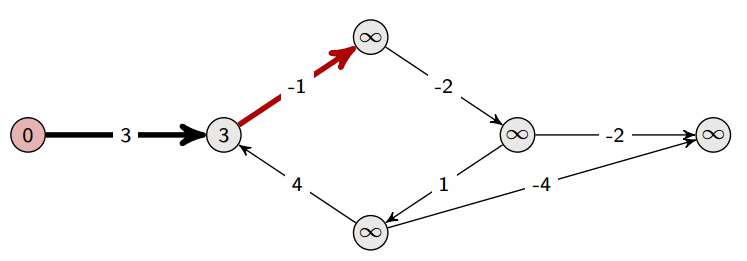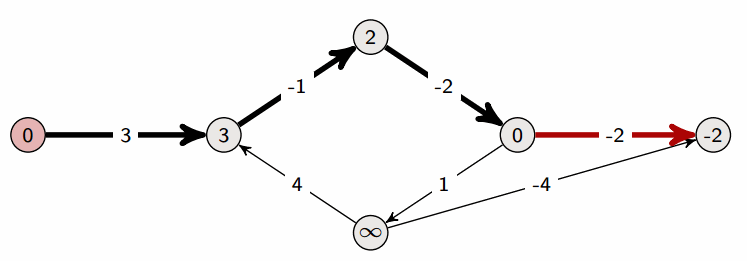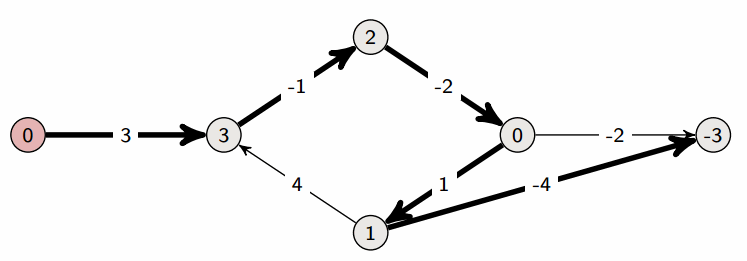
- 알고리즘이 시작할 땐 그래프의 구조를 모른다.
- 시작점에서 시작점까지 거리는 0이고 나머지는 아주 큰 수로 초기화 한다.
- relax 과정을 반복적으로 실행하며 최단 거리에 가까워 진다.
음수 사이클의 판정
- 음수사이클의 존재 여부를 판정정하려면 |V|-1번 모든 간선에 대한 relax를 시도하는 대신 |V|번 relax를 시도하면 된다.
- 그래프에 음수 사이클이 없다면 |V|-1 번만의 반복으로 모든 최단 거리를 찾아내기 때문에 마지막 반복의 완화는 전부 실패한다.
벨만-포트 알고리즘의 구현
// A Java program for Bellman-Ford's single source shortest path
// algorithm.
import java.util.*;
import java.lang.*;
import java.io.*;
// A class to represent a connected, directed and weighted graph
class Graph {
// A class to represent a weighted edge in graph
class Edge {
int src, dest, weight;
Edge()
{
src = dest = weight = 0;
}
};
int V, E;
Edge edge[];
// Creates a graph with V vertices and E edges
Graph(int v, int e)
{
V = v;
E = e;
edge = new Edge[e];
for (int i = 0; i < e; ++i)
edge[i] = new Edge();
}
// The main function that finds shortest distances from src
// to all other vertices using Bellman-Ford algorithm. The
// function also detects negative weight cycle
void BellmanFord(Graph graph, int src)
{
int V = graph.V, E = graph.E;
int dist[] = new int[V];
// Step 1: Initialize distances from src to all other
// vertices as INFINITE
for (int i = 0; i < V; ++i)
dist[i] = Integer.MAX_VALUE;
dist[src] = 0;
// Step 2: Relax all edges |V| - 1 times. A simple
// shortest path from src to any other vertex can
// have at-most |V| - 1 edges
for (int i = 1; i < V; ++i) {
for (int j = 0; j < E; ++j) {
int u = graph.edge[j].src;
int v = graph.edge[j].dest;
int weight = graph.edge[j].weight;
if (dist[u] != Integer.MAX_VALUE && dist[u] + weight < dist[v])
dist[v] = dist[u] + weight;
}
}
// Step 3: check for negative-weight cycles. The above
// step guarantees shortest distances if graph doesn't
// contain negative weight cycle. If we get a shorter
// path, then there is a cycle.
for (int j = 0; j < E; ++j) {
int u = graph.edge[j].src;
int v = graph.edge[j].dest;
int weight = graph.edge[j].weight;
if (dist[u] != Integer.MAX_VALUE && dist[u] + weight < dist[v]) {
System.out.println("Graph contains negative weight cycle");
return;
}
}
printArr(dist, V);
}
// A utility function used to print the solution
void printArr(int dist[], int V)
{
System.out.println("Vertex Distance from Source");
for (int i = 0; i < V; ++i)
System.out.println(i + "\t\t" + dist[i]);
}
// Driver method to test above function
public static void main(String[] args)
{
int V = 5; // Number of vertices in graph
int E = 8; // Number of edges in graph
Graph graph = new Graph(V, E);
// add edge 0-1 (or A-B in above figure)
graph.edge[0].src = 0;
graph.edge[0].dest = 1;
graph.edge[0].weight = -1;
// add edge 0-2 (or A-C in above figure)
graph.edge[1].src = 0;
graph.edge[1].dest = 2;
graph.edge[1].weight = 4;
// add edge 1-2 (or B-C in above figure)
graph.edge[2].src = 1;
graph.edge[2].dest = 2;
graph.edge[2].weight = 3;
// add edge 1-3 (or B-D in above figure)
graph.edge[3].src = 1;
graph.edge[3].dest = 3;
graph.edge[3].weight = 2;
// add edge 1-4 (or A-E in above figure)
graph.edge[4].src = 1;
graph.edge[4].dest = 4;
graph.edge[4].weight = 2;
// add edge 3-2 (or D-C in above figure)
graph.edge[5].src = 3;
graph.edge[5].dest = 2;
graph.edge[5].weight = 5;
// add edge 3-1 (or D-B in above figure)
graph.edge[6].src = 3;
graph.edge[6].dest = 1;
graph.edge[6].weight = 1;
// add edge 4-3 (or E-D in above figure)
graph.edge[7].src = 4;
graph.edge[7].dest = 3;
graph.edge[7].weight = -3;
graph.BellmanFord(graph, 0);
}
}
// Contributed by Aakash Hasija
플로이드의 모든 쌍 최단 거리 알고리즘
모든 쌍 간의 최단 거리를 구하고 싶을 때 사용
Resource
dijkstra.gif Source View,Pictures preview,Source program download - interviews
interviews Pictures show,dijkstra.gif Source code Your browser does not support the picture display, upgrade or replacement of the browser or the file to download to your computer to watch If your browser can not view dijkstra.gif,Please download to comput
www.bvbcode.com
Dijkstra Algorithm in Java
Dijkstra Algorithm in Java. GitHub Gist: instantly share code, notes, and snippets.
gist.github.com
Back to Basics — Divine Algorithms Vol II: Bellman-Ford Algorithm
A Shortest Path Expedition
medium.com
Bellman–Ford Algorithm | DP-23 - GeeksforGeeks
A Computer Science portal for geeks. It contains well written, well thought and well explained computer science and programming articles, quizzes and practice/competitive programming/company interview Questions.
www.geeksforgeeks.org
'Algorithm > Study' 카테고리의 다른 글
| 😈 Greedy & Prims Algorithm (feat MST) (0) | 2020.06.18 |
|---|---|
| 01. 배열과 문자열 (0) | 2020.06.09 |
| 문제해결 전략 - 29. 그래프 너비 우선 탐색 (0) | 2020.05.22 |
| 문제해결 전략 - 28. 그래프 깊이 우선 탐색 (0) | 2020.05.14 |
| 문제해결 전략 - 27. 그래프 표현과 정의 (0) | 2020.05.07 |



댓글